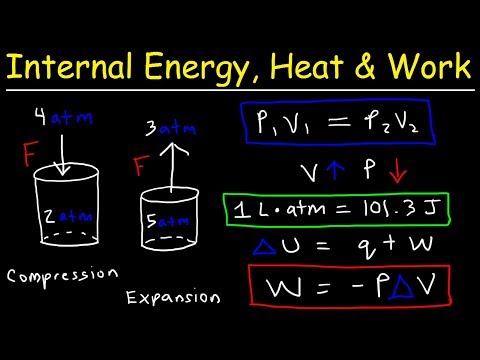
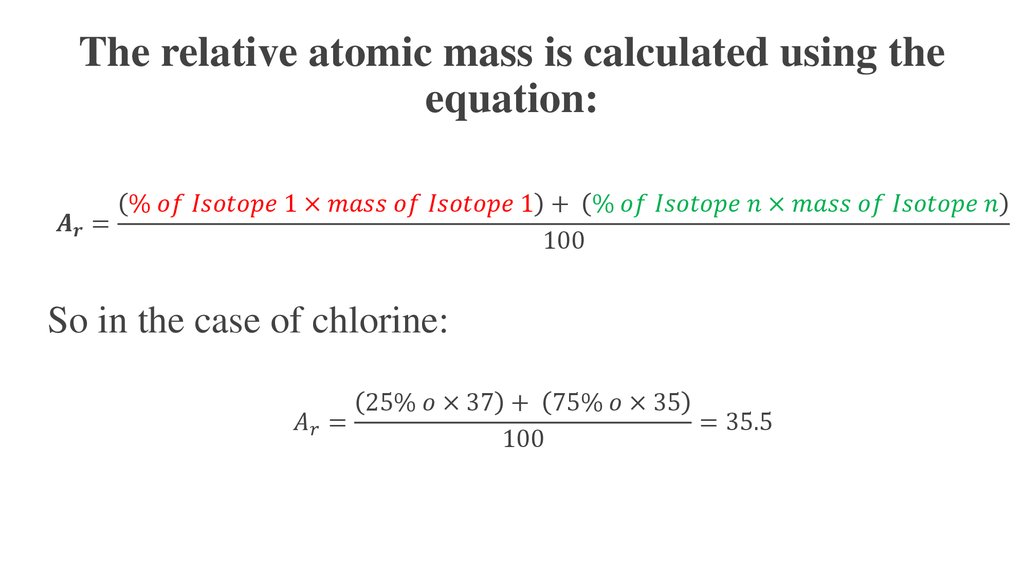
The ideal gas law can be used to calculate the volume of gaseous products or reactants as needed. A gas collected in such a way is not pure, however, but contains a significant amount of water vapor. The measured pressure must therefore be corrected for the vapor pressure of water, which depends strongly on the temperature. Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures.
We will consider the key developments in individual relationships , then put them together in the ideal gas law. The behavior of gases can be described by several laws based on experimental observations of their properties. The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons's law).
The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles's law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle's law). Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro's law). For more accurate measurements, glassware that has been certified by standards agencies may be purchased.
Table 3.2-2 lists the calculated volumes for one gram of water in air at atmospheric pressure at sea level for different temperatures, corrected for buoyancy with stainless steel weights of density 7.8 kg m−3. The glass volumes are also calculated for the standard temperature of 20 °C, with small adjustments for borosilicate glass expansion or contraction with temperature changes. To have value, measurement results must be metrologically traceable to an appropriate reference, which in the cases treated in this chapter are SI units of mass, volume, and amount of substance. A statement of measurement uncertainty always accompanies a traceable result. Methods must be validated and verified for use by a particular operator at a particular time.
Accreditation to an appropriate standard, such as ISO , is overseen by organisations usually with governmental or quasi-governmental status. Gaining accreditation for a particular method shows that a laboratory is using validated methods by competent personnel, but of course can never guarantee a reliable result . In this section we will review the components of measurement uncertainty of mass and volume measurements and then apply this to the preparation of a standard solution and a typical titration. It is noted that the metrological traceability chain will involve multiple branches , often through amount fraction or mass fraction. For more information, see the chapter on quality assurance in the forthcoming 4th edition of the Orange Book , or in . When products or reactants are gaseous in form at standard temperature and pressure, the molar volume of a gas and mole ratios can be used to convert the volume of one substance to moles of another substance.
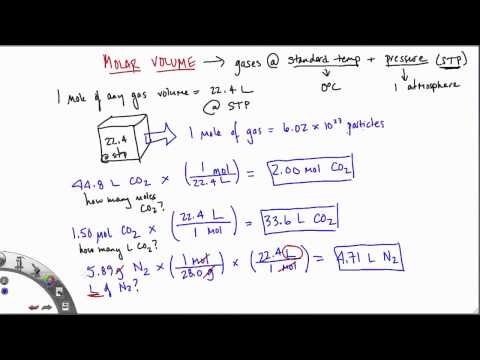
One mole of an ideal gas has a volume of 22.4 L at standard temperature and pressure. Avogadro was an Italian Physicist who first described the Avogadro constant as a hypothesis in 1811. He was trying to understand why in chemical reactions involving gases the observation that equal volumes of different gases had the same number of moles.
This was found true even when the masses were very different. The idea that a mole of any substance has exactly the same number of atoms no matter what the substance is made of was explained by Avogadro and his name has stuck to his number ever since. First convert this volume into mass using density (g/mL), then convert grams to moles using the molecular weight. Again, include units and set up your calculation so that milliliters and grams cancel in the calculation leaving an answer that has units of moles. Volume is the quantification of the three-dimensional space a substance occupies. By convention, the volume of a container is typically its capacity, and how much fluid it is able to hold, rather than the amount of space that the actual container displaces.
Volumes of many shapes can be calculated by using well-defined formulas. In some cases, more complicated shapes can be broken down into simpler aggregate shapes, and the sum of their volumes is used to determine total volume. The volumes of other even more complicated shapes can be calculated using integral calculus if a formula exists for the shape's boundary. Beyond this, shapes that cannot be described by known equations can be estimated using mathematical methods, such as the finite element method.
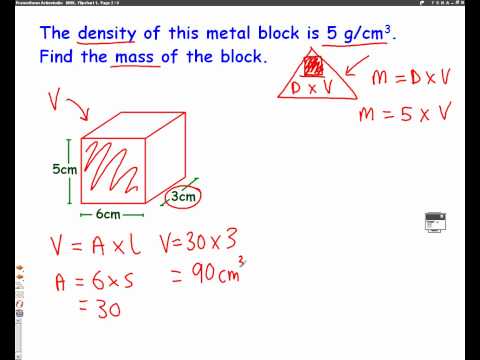
Alternatively, if the density of a substance is known, and is uniform, the volume can be calculated using its weight. This calculator computes volumes for some of the most common simple shapes. This method is useful to calculate the volume of irregular solids, for eg, cone, cylinder, etc.
For regular three-dimensional objects, you can easily calculate the volume by taking measurements of its dimensions and applying the appropriate volume equation. If it's an irregular shape, you can try to do the very thing that caused Archimedes to shout the famous word Eureka! Probably you heard that story - Archimedes was asked to find out if the Hiero's crown is made from pure gold or just gold-plated - but without bending or destroying it. The idea came to him when he was taking a bath - stepping into a bathtub, he noticed that the water level rose. From this observation, he deduced that volume of water displaced must be equal to the volume of the part of his body he had submerged.
Knowing the irregular object volume and its weight, he could calculate the density and compare it with the density of pure gold. Legend says that Archimedes was so excited about this discovery that he popped out of his bathtub and ran naked through the streets of Syracuse. Specific volume is defined as the number of cubic meters occupied by one kilogram of matter.
It is the ratio of a material's volume to its mass, which is the same as the reciprocal of its density. In other words, specific volume is inversely proportional to density. Specific volume may be calculated or measured for any state of matter, but it is most often used in calculations involving gases. Gases whose properties of P, V, and T are accurately described by the ideal gas law are said to exhibit ideal behavior or to approximate the traits of an ideal gas. An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter.
Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature. In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures. Measuring the volume depends on your object's state of matter. For liquids, you can use a graduated cylinder or burette for the chemistry lab measurements, or a measuring cup & spoon for everyday life purposes.
For gases, to roughly measure the volume, you can inflate a balloon and use it to displace the water in a graduate cylinder. A similar method works for solids — put the object into a graduated container and measure the change in reading. Is the volume occupied by one mole of a chemical element or a chemical compound. It can be calculated by dividing the molar mass by mass density (ρ). Molar gas volume is one mole of any gas at a specific temperature and pressure has a fixed volume. The relative formula mass of a compound is calculated by adding together the relative atomic mass values for all the atoms in its formula.
It is important to note that the molarity is defined as moles of solute per liter of solution, not moles of solute per liter of solvent. This is because when you add a substance, perhaps a salt, to some volume of water, the volume of the resulting solution will be different than the original volume in some unpredictable way. To get around this problem chemists commonly make up their solutions in volumetric flasks. These are flasks that have a long neck with an etched line indicating the volume. The solute is added to the flask first and then water is added until the solution reaches the mark.
The flasks have very good calibration so volumes are commonly known to at least four significant figures. In thermodynamics, the volume of a system is an important extensive parameter for describing its thermodynamic state. The specific volume, an intensive property, is the system's volume per unit of mass.
Volume is a function of state and is interdependent with other thermodynamic properties such as pressure and temperature. For example, volume is related to the pressure and temperature of an ideal gas by the ideal gas law. For example, the space that a substance or 3D shape occupies or contains. Volume is often quantified numerically using the SI derived unit, the cubic metre.
Volumes of some simple shapes, such as regular, straight-edged, and circular shapes can be easily calculated using arithmetic formulas. Volumes of complicated shapes can be calculated with integral calculus if a formula exists for the shape's boundary. One-dimensional figures and two-dimensional shapes are assigned zero volume in the three-dimensional space. End-point error – the systematic error occurring because the equivalence-point potential differs from the end-point potential under the given conditions of titration. The equivalence-point potential depends on the formal potentials of the analyte and titrant and on the number of electrons participating in half-reactions. When the transition potential, corresponding to the end-point, is close to the equivalence-point potential, the effect of the above-mentioned factors may be diminished.
In fact, gravimetric analysis was used to determine the atomic masses of many elements to six-figure accuracy. The measurement of mass is a central point of the quantification of material substances. A balance measures mass by sensing the weight force that presses an object down on the balance pan. Weight is the force exerted on a body by the gravitational field of the earth, and is measured in the unit force newton, N. The weight force acting on 1 kg mass depends on geographic and cosmic factors. However, for mass measurements using mechanical balances, the weight of the unknown object is equilibrated at the same place and same time as the weight of an object of known mass (i.e. of a standard).
For high precision measurements, the buoyancy caused by the surrounding air must be taken into consideration. This correction can easily be calculated if the density of the known and unknown mass and that of the air is known. • To convert volume to moles, first convert to mass using density, then convert to moles using molecular weight. Again, be sure to include all units in your calculations.
The volume calculator will calculate the volume of some of the most common three-dimensional solids. Before we go into how to calculate volume, you must know the definition of volume. Volume differs from the area, which is the amount of space taken up in a two-dimensional figure. So you might be confused as to how to find the volume of a rectangle versus how to find the volume of a box. The calculator will assist in calculating the volume of a sphere, cylinder, cube, cone, and rectangular solids. We can calculate how many moles of a substance we have by dividing the mass in grams of a substance by molar mass .
Mr is the exact same thing as the relative formula mass. Specific volume is a property of matter that is its ratio of volume to mass or reciprocal of its density.Specific volume is a physical property of a substance that is the ratio of its volume to its mass. Specific volume applies to all states or matter, but it finds practical application for calculations involving gases.
We can use the ideal gas equation to calculate the volume of 1 mole of an ideal gas at 0°C and 1 atmosphere pressure. Standard solutions are often prepared by dissolving an accurately measured mass of a solute of certified purity in a known volume of solvent. If the concentration of an intended standard solution is obtained by measurement, for example by titration with a standard solution, it is known as a secondary standard [VIM 5.5]. Add the atomic masses of the solute together to find the molar mass. Look at the elements in the chemical formula for the solute you're using. List the atomic mass for each element in the solute since atomic and molar mass are the same.
Add together the atomic masses from your solute to find the total molar mass. Transition potential is often given instead of the formal redox potential. It corresponds to the colour change at which the end-point is said to occur. It is a function of the formal redox potential, the total concentration of the indicator , the depth of the colour layer, the minimal observable absorbance , and the absorption coefficient.
In an ideal two-colour indicator, the "apparent absorption coefficients" of both forms should be equal. Then the transition potential approaches the formal one. As for formal redox potential, it should be given, at least for the acidity range of indicator application.
The transition potential may be given for pseudo-reversible indicators. Because the transition point is usually different for oxidimetric and reductiometric titrations, it is sometimes useful to distinguish those two values. This means equal amounts of moles of gases occupy the same volume under the same conditions of temperature and pressure. Examples and practice problems of solving equation stoichiometry questions with gases. We calculate moles with 22.4 L at STP, and use molar mass and mole ratios to figure out how many products or reactants we have. The most common molar volume is the molar volume of an ideal gas at standard temperature and pressure (273 K and 1.00 atm).
And even to estimate molecular weights, and there are many reports of such studies in the literature, as highlighted in the following section. A small teaspoon of sodium hydrogencarbonate weighs 4.2g. Calculate the moles, mass and volume of carbon dioxide formed when it is thermally decomposed in the oven.
Assume room temperature for the purpose of the calculation. • Mass can be converted to moles using molecular weight. By insuring that the mass units cancel in the calculation you can be sure you have the calculation setup properly. Because many objects are not regularly shaped their volume cannot be determined using a volume formula. The volume of these objects can be found by water displacement. A volume of water sufficient to cover the object is placed in a graduated cylinder and the volume read.
The object is added to the cylinder and the volume read again. The difference between the two volumes is the volume of the object. This method is demonstrated using the same battery used above. An aqueous solution consists of at least two components, the solvent and the solute . Usually one wants to keep track of the amount of the solute dissolved in the solution.
One could do by keeping track of the concentration by determining the mass of each component, but it is usually easier to measure liquids by volume instead of mass. Molarity is defined as the number of moles of solute divided by the volume of the solution in liters. This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in Figure 9.11. The volume and temperature are linearly related for 1 mole of methane gas at a constant pressure of 1 atm.